Always-On Quantum Error Tracking with Continuous Parity Measurements

Razieh Mohseninia; Jing Yang; Irfan Siddiqi; Andrew N. Jordan; Justin Dressel

doi:10.22331/q-2020-11-04-358

Always-On Quantum Error Tracking with Continuous Parity Measurements

Razieh Mohseninia^1,2, Jing Yang³, Irfan Siddiqi^4,5, Andrew N. Jordan^3,1, and Justin Dressel^1,6

¹Institute for Quantum Studies, Chapman University, Orange, CA 92866, USA
²Departments of Electrical Engineering, University of Southern California, Los Angeles, California 90089, USA
³Department of Physics and Astronomy, University of Rochester, Rochester, New York 14627, USA
⁴Center for Quantum Coherent Science, Berkeley, CA 94720 USA
⁵Department of Physics, University of California, Berkeley, CA 94720 USA
⁶Schmid College of Science and Technology, Chapman University, Orange, CA 92866, USA

Published:	2020-11-04, volume 4, page 358
Eprint:	arXiv:1907.08882v2
Doi:	https://doi.org/10.22331/q-2020-11-04-358
Citation:	Quantum 4, 358 (2020).

Find this paper interesting or want to discuss? Scite or leave a comment on SciRate.

Abstract

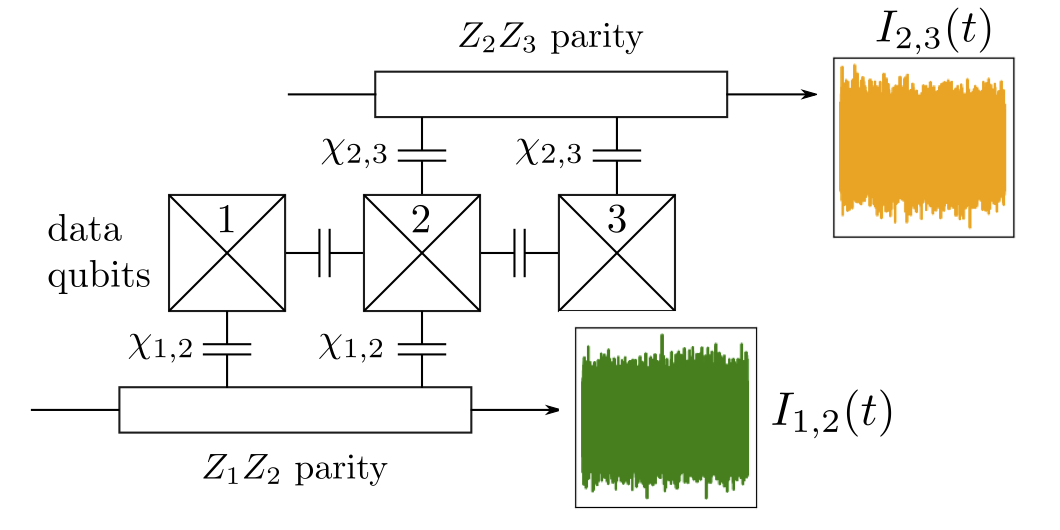
We investigate quantum error correction using continuous parity measurements to correct bit-flip errors with the three-qubit code. Continuous monitoring of errors brings the benefit of a continuous stream of information, which facilitates passive error tracking in real time. It reduces overhead from the standard gate-based approach that periodically entangles and measures additional ancilla qubits. However, the noisy analog signals from continuous parity measurements mandate more complicated signal processing to interpret syndromes accurately. We analyze the performance of several practical filtering methods for continuous error correction and demonstrate that they are viable alternatives to the standard ancilla-based approach. As an optimal filter, we discuss an unnormalized (linear) Bayesian filter, with improved computational efficiency compared to the related Wonham filter introduced by Mabuchi [New J. Phys. 11, 105044 (2009)]. We compare this optimal continuous filter to two practical variations of the simplest periodic boxcar-averaging-and-thresholding filter, targeting real-time hardware implementations with low-latency circuitry. As variations, we introduce a non-Markovian ``half-boxcar'' filter and a Markovian filter with a second adjustable threshold; these filters eliminate the dominant source of error in the boxcar filter, and compare favorably to the optimal filter. For each filter, we derive analytic results for the decay in average fidelity and verify them with numerical simulations.

Popular summary

► BibTeX data

@article{Mohseninia2020alwaysquantumerror,
  doi = {10.22331/q-2020-11-04-358},
  url = {https://doi.org/10.22331/q-2020-11-04-358},
  title = {Always-{O}n {Q}uantum {E}rror {T}racking with {C}ontinuous {P}arity {M}easurements},
  author = {Mohseninia, Razieh and Yang, Jing and Siddiqi, Irfan and Jordan, Andrew N. and Dressel, Justin},
  journal = {{Quantum}},
  issn = {2521-327X},
  publisher = {{Verein zur F{\"{o}}rderung des Open Access Publizierens in den Quantenwissenschaften}},
  volume = {4},
  pages = {358},
  month = nov,
  year = {2020}
}

► References

[1] D. A. Lidar and T. A. Brun. Quantum error correction. Cambridge University Press, 2013. 10.1017/CBO9781139034807.
https://doi.org/10.1017/CBO9781139034807

[2] F. Gaitan. Quantum Error Correction and Fault Tolerant Quantum Computing. Boca Raton: CRC Press, 2013. ISBN 9781315221885. 10.1201/b15868.
https://doi.org/10.1201/b15868

[3] M. H. Devoret, A. Wallraff, and J. M. Martinis. Superconducting qubits: A short review. arXiv preprint cond-mat/0411174, 2004. URL https://arxiv.org/abs/cond-mat/0411174.
https://arxiv.org/abs/cond-mat/0411174

[4] M. H. Devoret and R. J. Schoelkopf. Superconducting circuits for quantum information: An outlook. Science, 339 (6124): 1169–1174, 2013. 10.1126/science.1231930.
https://doi.org/10.1126/science.1231930

[5] M. A. Nielsen and I. L. Chuang. Quantum Computation and Quantum Information. Cambridge University Press, New York, NY, USA, 10th edition, 2010. 10.1017/cbo9780511976667.
https://doi.org/10.1017/cbo9780511976667

[6] P. W. Shor. Scheme for reducing decoherence in quantum computer memory. Physical Review A, 52 (4): R2493–R2496, October 1995. 10.1103/PhysRevA.52.R2493.
https://doi.org/10.1103/PhysRevA.52.R2493

[7] A. M. Steane. Error correcting codes in quantum theory. Physical Review Letters, 77 (5): 793–797, 1996a. 10.1103/PhysRevLett.77.793.
https://doi.org/10.1103/PhysRevLett.77.793

[8] A. R. Calderbank and P. W. Shor. Good quantum error-correcting codes exist. Physical Review A, 54 (2): 1098–1105, 1996. 10.1103/PhysRevA.54.1098.
https://doi.org/10.1103/PhysRevA.54.1098

[9] A. M. Steane. Simple quantum error-correcting codes. Physical Review A, 54 (6): 4741, 1996b. 10.1103/PhysRevA.54.4741.
https://doi.org/10.1103/PhysRevA.54.4741

[10] D. Gottesman. Class of quantum error-correcting codes saturating the quantum Hamming bound. Physical Review A, 54 (3): 1862–1868, 1996. 10.1103/PhysRevA.54.1862.
https://doi.org/10.1103/PhysRevA.54.1862

[11] D. Gottesman. Stabilizer Codes and Quantum Error Correction. arXiv preprint quant-ph/9705052, May 1997. URL https://arxiv.org/abs/quant-ph/9705052.
arXiv:quant-ph/9705052

[12] D. Gottesman. Theory of fault-tolerant quantum computation. Physical Review A, 57 (1): 127–137, 1998. 10.1103/PhysRevA.57.127.
https://doi.org/10.1103/PhysRevA.57.127

[13] D. Gottesman. An introduction to quantum error correction and fault-tolerant quantum computation. arXiv preprint arXiv:0904.2557, april 2009. URL https://arxiv.org/abs/0904.2557.
arXiv:0904.2557

[14] A. Chowdhury and B. S. Rajan. Quantum error correction via codes over GF (2). IEEE International Symposium on Information Theory - Proceedings, 44 (4): 789–793, 2009. 10.1109/ISIT.2009.5205646.
https://doi.org/10.1109/ISIT.2009.5205646

[15] M. D. Reed, L. Dicarlo, S. E. Nigg, L. Sun, L. Frunzio, S. M. Girvin, and R. J. Schoelkopf. Realization of three-qubit quantum error correction with superconducting circuits. Nature, 482 (7385): 382–385, February 2012. 10.1038/nature10786.
https://doi.org/10.1038/nature10786

[16] R. Barends, J. Kelly, A. Megrant, A. Veitia, D. Sank, E. Jeffrey, T. C. White, J. Mutus, A. G. Fowler, B. Campbell, Y. Chen, Z. Chen, B. Chiaro, A. Dunsworth, C. Neill, P. O'Malley, P. Roushan, A. Vainsencher, J. Wenner, A. N. Korotkov, A. N. Cleland, and J. M. Martinis. Superconducting quantum circuits at the surface code threshold for fault tolerance. Nature, 508 (7497): 500–503, April 2014. 10.1038/nature13171.
https://doi.org/10.1038/nature13171

[17] J. M. Chow, J. M. Gambetta, E. Magesan, D. W. Abraham, A. W. Cross, B. R. Johnson, N. A. Masluk, C. A. Ryan, J. A. Smolin, S. J. Srinivasan, and M. Steffen. Implementing a strand of a scalable fault-tolerant quantum computing fabric. Nature Communications, 5: ncomms5015, June 2014. 10.1038/ncomms5015.
https://doi.org/10.1038/ncomms5015

[18] I. Siddiqi, S. J. Weber, K. W. Murch, A. Chantasri, J. Dressel, and A. N. Jordan. Observing single quantum trajectories of a superconducting qubit. Optics InfoBase Conference Papers, 502 (7470): 211, 2014. 10.1364/qim.2014.qw1a.1.
https://doi.org/10.1364/qim.2014.qw1a.1

[19] S. J. Weber, A. Chantasri, J. Dressel, A. N. Jordan, K. W. Murch, and I. Siddiqi. Mapping the optimal route between two quantum states. Nature, 511 (7511): 570–573, 2014. 10.1038/nature13559.
https://doi.org/10.1038/nature13559

[20] Q. Ficheux, S. Jezouin, P. Campagne-Ibarcq, P. Rouchon, and B. Huard. Observing quantum trajectories based on the fluorescence and dispersive measurement records of a superconducting qubit. In Optics InfoBase Conference Papers, volume Part F73-Q, pages QF5A—-2. Optical Society of America, 2017. ISBN 9781557528209. 10.1364/QIM.2017.QF5A.2.
https://doi.org/10.1364/QIM.2017.QF5A.2

[21] P. Campagne-Ibarcq, P. Six, L. Bretheau, A. Sarlette, M. Mirrahimi, P. Rouchon, and B. Huard. Observing quantum state diffusion by heterodyne detection of fluorescence. Physical Review X, 6 (1): 11002, 2016. 10.1103/PhysRevX.6.011002.
https://doi.org/10.1103/PhysRevX.6.011002

[22] H. M. Wiseman and G. J. Milburn. Quantum measurement and control, volume 9780521804. Cambridge University Press, Cambridge, 2009. ISBN 9780511813948. 10.1017/CBO9780511813948.
https://doi.org/10.1017/CBO9780511813948

[23] K. Jacobs. Quantum measurement theory and its applications. Cambridge University Press, August 2014. ISBN 9781139992190. 10.1017/CBO9781139179027.
https://doi.org/10.1017/CBO9781139179027

[24] W. Mao, D. V. Averin, R. Ruskov, and A. N. Korotkov. Mesoscopic quadratic quantum measurements. Physical Review Letters, 93 (5): 56803, 2004. 10.1103/PhysRevLett.93.056803.
https://doi.org/10.1103/PhysRevLett.93.056803

[25] B. Trauzettel, A. N. Jordan, C. W. Beenakker, and M. Büttiker. Parity meter for charge qubits: An efficient quantum entangler. Physical Review B, 73 (23): 235331, 2006. 10.1103/PhysRevB.73.235331.
https://doi.org/10.1103/PhysRevB.73.235331

[26] N. S. Williams and A. N. Jordan. Entanglement genesis under continuous parity measurement. Physical Review A, 78 (6): 62322, 2008. 10.1103/PhysRevA.78.062322.
https://doi.org/10.1103/PhysRevA.78.062322

[27] K. Lalumière, J. M. Gambetta, and A. Blais. Tunable joint measurements in the dispersive regime of cavity QED. Physical Review A, 81 (4): 40301, 2010. 10.1103/PhysRevA.81.040301.
https://doi.org/10.1103/PhysRevA.81.040301

[28] L. Tornberg and G. Johansson. High-fidelity feedback-assisted parity measurement in circuit QED. Physical Review A - Atomic, Molecular, and Optical Physics, 82 (1): 12329, 2010. 10.1103/PhysRevA.82.012329.
https://doi.org/10.1103/PhysRevA.82.012329

[29] G. Haack, H. Förster, and M. Büttiker. Parity detection and entanglement with a Mach-Zehnder interferometer. Physical Review B, 82 (15): 155303, 2010. 10.1103/PhysRevB.82.155303.
https://doi.org/10.1103/PhysRevB.82.155303

[30] C. Meyer Zu Rheda, G. Haack, and A. Romito. On-demand maximally entangled states with a parity meter and continuous feedback. Physical Review B, 90 (15): 155438, 2014. 10.1103/PhysRevB.90.155438.
https://doi.org/10.1103/PhysRevB.90.155438

[31] C. Ahn, A. C. Doherty, and A. J. Landahl. Continuous quantum error correction via quantum feedback control. Physical Review A, 65 (4): 10, March 2002. 10.1103/PhysRevA.65.042301.
https://doi.org/10.1103/PhysRevA.65.042301

[32] C. Ahn, H. M. Wiseman, and G. J. Milburn. Quantum error correction for continuously detected errors. Physical Review A, 67 (5): 11, May 2003. 10.1103/PhysRevA.67.052310.
https://doi.org/10.1103/PhysRevA.67.052310

[33] M. Sarovar, C. Ahn, K. Jacobs, and G. J. Milburn. Practical scheme for error control using feedback. Physical Review A, 69 (5 A): 52324, May 2004. 10.1103/PhysRevA.69.052324.
https://doi.org/10.1103/PhysRevA.69.052324

[34] R. van Handel and H. Mabuchi. Optimal error tracking via quantum coding and continuous syndrome measurement. arXiv preprint quant-ph/0511221, November 2005. URL http://arxiv.org/abs/quant-ph/0511221.
arXiv:quant-ph/0511221

[35] H. Mabuchi. Continuous quantum error correction as classical hybrid control. New Journal of Physics, 11 (10): 105044, 2009. 10.1088/1367-2630/11/10/105044.
https://doi.org/10.1088/1367-2630/11/10/105044

[36] G. Denhez, A. Blais, and D. Poulin. Quantum-error-correction benchmarks for continuous weak-parity measurements. Physical Review A, 86 (3): 32318, 2012. 10.1103/PhysRevA.86.032318.
https://doi.org/10.1103/PhysRevA.86.032318

[37] K.-C. Hsu and T. A. Brun. Method for quantum-jump continuous-time quantum error correction. Physical Review A, 93 (2): 22321, February 2016. 10.1103/PhysRevA.93.022321.
https://doi.org/10.1103/PhysRevA.93.022321

[38] J. Atalaya, A. N. Korotkov, and K. B. Whaley. Error-correcting Bacon-Shor code with continuous measurement of noncommuting operators. Physical Review A, 102 (2): 32317, 2020. 10.1103/PhysRevA.102.022415.
https://doi.org/10.1103/PhysRevA.102.022415

[39] G. Cardona, A. Sarlette, and P. Rouchon. Continuous-time quantum error correction with noise-assisted quantum feedback. IFAC-PapersOnLine, 52 (16): 198–203, 2019. 10.1016/j.ifacol.2019.11.778.
https://doi.org/10.1016/j.ifacol.2019.11.778

[40] D. Ristè, M. Dukalski, C. A. Watson, G. De Lange, M. J. Tiggelman, Y. M. Blanter, K. W. Lehnert, R. N. Schouten, and L. Dicarlo. Deterministic entanglement of superconducting qubits by parity measurement and feedback. Nature, 502 (7471): 350–354, 2013. 10.1038/nature12513.
https://doi.org/10.1038/nature12513

[41] N. Roch, M. E. Schwartz, F. Motzoi, C. Macklin, R. Vijay, A. W. Eddins, A. N. Korotkov, K. B. Whaley, M. Sarovar, and I. Siddiqi. Observation of measurement-induced entanglement and quantum trajectories of remote superconducting qubits. Physical Review Letters, 112 (17): 170501, April 2014. 10.1103/PhysRevLett.112.170501.
https://doi.org/10.1103/PhysRevLett.112.170501

[42] A. Chantasri, M. E. Kimchi-Schwartz, N. Roch, I. Siddiqi, and A. N. Jordan. Quantum trajectories and their statistics for remotely entangled quantum bits. Physical Review X, 6 (4): 41052, December 2016. 10.1103/PhysRevX.6.041052.
https://doi.org/10.1103/PhysRevX.6.041052

[43] A. Narla, S. Shankar, M. Hatridge, Z. Leghtas, K. M. Sliwa, E. Zalys-Geller, S. O. Mundhada, W. Pfaff, L. Frunzio, R. J. Schoelkopf, and M. H. Devoret. Robust concurrent remote entanglement between two superconducting qubits. Physical Review X, 6 (3): 31036, September 2016. 10.1103/PhysRevX.6.031036.
https://doi.org/10.1103/PhysRevX.6.031036

[44] P. Kumar and A. Patel. Quantum error correction using weak measurements. Quantum Information Processing, 18 (2): 58, 2019. 10.1007/s11128-018-2166-5.
https://doi.org/10.1007/s11128-018-2166-5

[45] W. Livingston, M. Blok, E. Flurin, J. Atalaya, J. Dressel, A. Jordan, A. Korotkov, and I. Siddiqi. Implementation of Continuous Parity Measurements and Error Correction. In APS Meeting Abstracts, page A39.011, 2018.

[46] W. Livingston, M. Blok, J. Atalaya, J. Yang, R. Mohseninia, A. Jordan, J. Dressel, A. Korotkov, and I. Siddiqi. Continuous Parity Measurement and Error Correction. In APS Meeting Abstracts, page L27.002, 2019.

[47] W. Zucchini, I. L. MacDonald, and R. Langrock. Hidden Markov models for time series: an introduction using R. CRC press, 2017. ISBN 9781482253849. 10.1201/b20790.
https://doi.org/10.1201/b20790

[48] M. Gales and S. Young. The application of hidden markov models in speech recognition. Found. Trends Signal Process., 1 (3): 195–304, January 2007. 10.1561/2000000004.
https://doi.org/10.1561/2000000004

[49] J. Koch, T. M. Yu, J. Gambetta, A. A. Houck, D. I. Schuster, J. Majer, A. Blais, M. H. Devoret, S. M. Girvin, and R. J. Schoelkopf. Charge-insensitive qubit design derived from the Cooper pair box. Physical Review A, 76 (4): 42319, 2007. 10.1103/PhysRevA.76.042319.
https://doi.org/10.1103/PhysRevA.76.042319

[50] D. H. Slichter, C. Müller, R. Vijay, S. J. Weber, A. Blais, and I. Siddiqi. Quantum Zeno effect in the strong measurement regime of circuit quantum electrodynamics. New Journal of Physics, 18 (5): 53031, 2016. 10.1088/1367-2630/18/5/053031.
https://doi.org/10.1088/1367-2630/18/5/053031

[51] J. J. Faraway and J. S. Simonoff. Smoothing Methods in Statistics, volume 92. Springer-Verlag: New York, 1997. 10.2307/2965589.
https://doi.org/10.2307/2965589

[52] C. J. Ellison, J. R. Mahoney, and J. P. Crutchfield. Prediction, retrodiction, and the amount of information stored in the present. Journal of Statistical Physics, 136 (6): 1005–1034, 2009. 10.1007/s10955-009-9808-z.
https://doi.org/10.1007/s10955-009-9808-z

[53] G. A. Einicke. Smoothing, Filtering and Prediction - Estimating The Past, Present and Future. InTech, 2012. 10.5772/2706.
https://doi.org/10.5772/2706

[54] W. M. Wonham. Some applications of stochastic differential equations to optimal nonlinear filtering. Journal of the Society for Industrial and Applied Mathematics, Series A: Control, 2 (3): 347–369, 1964. 10.1137/0302028.
https://doi.org/10.1137/0302028

[55] K. Vahala. Handbook of stochastic methods for physics, chemistry and the natural sciences. IEEE Journal of Quantum Electronics, 22 (9): 1922–1922, 2004. 10.1109/jqe.1986.1073148.
https://doi.org/10.1109/jqe.1986.1073148

[56] W. J. Tsay, C. J. Huang, T. T. Fu, and I. L. Ho. A simple closed-form approximation for the cumulative distribution function of the composite error of stochastic frontier models. Journal of Productivity Analysis, 39 (3): 259–269, June 2013. 10.1007/s11123-012-0283-1.
https://doi.org/10.1007/s11123-012-0283-1

[57] M. Tomasi and M. Giordano. Towards new solutions for scientific computing: the case of Julia. arXiv preprint arXiv:1812.01219, 2018. URL http://arxiv.org/abs/1812.01219.
arXiv:1812.01219

[58] B. Criger, A. Ciani, and D. P. Divincenzo. Multi-qubit joint measurements in circuit QED: Stochastic master equation analysis. EPJ Quantum Technology, 3 (1): 6, 2016. 10.1140/epjqt/s40507-016-0044-6.
https://doi.org/10.1140/epjqt/s40507-016-0044-6

[59] A. Ciani and D. P. DiVincenzo. Three-qubit direct dispersive parity measurement with tunable coupling qubits. Physical Review B, 96 (21): 214511, 2017. 10.1103/PhysRevB.96.214511.
https://doi.org/10.1103/PhysRevB.96.214511

[60] P. Huembeli and S. E. Nigg. Towards a heralded eigenstate-preserving measurement of multi-qubit parity in circuit QED. Physical Review A, 96 (1): 12313, 2017. 10.1103/PhysRevA.96.012313.
https://doi.org/10.1103/PhysRevA.96.012313

[61] B. Royer, S. Puri, and A. Blais. Qubit parity measurement by parametric driving in circuit QED. Science Advances, 4 (11): eaau1695, 2018. 10.1126/sciadv.aau1695.
https://doi.org/10.1126/sciadv.aau1695

[62] J. M. Chow, A. D. Córcoles, J. M. Gambetta, C. Rigetti, B. R. Johnson, J. A. Smolin, J. R. Rozen, G. A. Keefe, M. B. Rothwell, M. B. Ketchen, and M. Steffen. Simple all-microwave entangling gate for fixed-frequency superconducting qubits. Physical Review Letters, 107 (8): 80502, 2011. 10.1103/PhysRevLett.107.080502.
https://doi.org/10.1103/PhysRevLett.107.080502

[63] S. Sheldon, E. Magesan, J. M. Chow, and J. M. Gambetta. Procedure for systematically tuning up cross-talk in the cross-resonance gate. Physical Review A, 93 (6): 60302, 2016. 10.1103/PhysRevA.93.060302.
https://doi.org/10.1103/PhysRevA.93.060302

[64] E. Magesan and J. M. Gambetta. Effective Hamiltonian models of the cross-resonance gate. Physical Review A, 101 (5): 052308, 2020. 10.1103/PhysRevA.101.052308.
https://doi.org/10.1103/PhysRevA.101.052308

[65] V. Tripathi, M. Khezri, and A. N. Korotkov. Operation and intrinsic error budget of a two-qubit cross-resonance gate. Physical Review A, 100 (1): 12301, 2019. 10.1103/PhysRevA.100.012301.
https://doi.org/10.1103/PhysRevA.100.012301

[66] A. D. Córcoles, E. Magesan, S. J. Srinivasan, A. W. Cross, M. Steffen, J. M. Gambetta, and J. M. Chow. Demonstration of a quantum error detection code using a square lattice of four superconducting qubits. Nature Communications, 6: 6979, 2015. 10.1038/ncomms7979.
https://doi.org/10.1038/ncomms7979

Cited by

[1] Philippe Lewalle, Cyril Elouard, and Andrew N. Jordan, "Entanglement-preserving limit cycles from sequential quantum measurements and feedback", Physical Review A 102 6, 062219 (2020).

[2] Sangkha Borah, Bijita Sarma, Michael Kewming, Fernando Quijandría, Gerard J. Milburn, and Jason Twamley, "Measurement-based estimator scheme for continuous quantum error correction", Physical Review Research 4 3, 033207 (2022).

[3] J. Atalaya, S. Zhang, M. Y. Niu, A. Babakhani, H. C. H. Chan, J. M. Epstein, and K. B. Whaley, "Continuous quantum error correction for evolution under time-dependent Hamiltonians", Physical Review A 103 4, 042406 (2021).

[4] G. Koolstra, N. Stevenson, S. Barzili, L. Burns, K. Siva, S. Greenfield, W. Livingston, A. Hashim, R. K. Naik, J. M. Kreikebaum, K. P. O’Brien, D. I. Santiago, J. Dressel, and I. Siddiqi, "Monitoring Fast Superconducting Qubit Dynamics Using a Neural Network", Physical Review X 12 3, 031017 (2022).

[5] Craig Gidney, Michael Newman, Austin Fowler, and Michael Broughton, "A Fault-Tolerant Honeycomb Memory", Quantum 5, 605 (2021).

[6] Ian Convy, Haoran Liao, Song Zhang, Sahil Patel, William P Livingston, Ho Nam Nguyen, Irfan Siddiqi, and K Birgitta Whaley, "Machine learning for continuous quantum error correction on superconducting qubits", New Journal of Physics 24 6, 063019 (2022).

[7] William P. Livingston, Machiel S. Blok, Emmanuel Flurin, Justin Dressel, Andrew N. Jordan, and Irfan Siddiqi, "Experimental demonstration of continuous quantum error correction", Nature Communications 13 1, 2307 (2022).

[8] Sacha Greenfield, Leigh Martin, Felix Motzoi, K. Birgitta Whaley, Justin Dressel, and Eli M. Levenson-Falk, "Stabilizing two-qubit entanglement with dynamically decoupled active feedback", Physical Review Applied 21 2, 024022 (2024).

[9] Ian Convy and K. Birgitta Whaley, "A Logarithmic Bayesian Approach to Quantum Error Detection", Quantum 6, 680 (2022).

[10] Juan Atalaya, Alexander N. Korotkov, and K. Birgitta Whaley, "Error-correcting Bacon-Shor code with continuous measurement of noncommuting operators", Physical Review A 102 2, 022415 (2020).

[11] Gregory Reznik, Shrobona Bagchi, Justin Dressel, and Lev Vaidman, "Footprints of quantum pigeons", Physical Review Research 2 2, 023004 (2020).

The above citations are from Crossref's cited-by service (last updated successfully 2024-05-12 06:50:15) and SAO/NASA ADS (last updated successfully 2024-05-12 06:50:15). The list may be incomplete as not all publishers provide suitable and complete citation data.

This Paper is published in Quantum under the Creative Commons Attribution 4.0 International (CC BY 4.0) license. Copyright remains with the original copyright holders such as the authors or their institutions.