Additivity of entropic uncertainty relations

René Schwonnek

doi:10.22331/q-2018-03-30-59

Additivity of entropic uncertainty relations

René Schwonnek

Institut für Theoretische Physik, Leibniz Universität Hannover, Germany

Published:	2018-03-30, volume 2, page 59
Eprint:	arXiv:1801.04602v4
Doi:	https://doi.org/10.22331/q-2018-03-30-59
Citation:	Quantum 2, 59 (2018).

Find this paper interesting or want to discuss? Scite or leave a comment on SciRate.

Abstract

We consider the uncertainty between two pairs of local projective measurements performed on a multipartite system. We show that the optimal bound in any linear uncertainty relation, formulated in terms of the Shannon entropy, is additive. This directly implies, against naive intuition, that the minimal entropic uncertainty can always be realized by fully separable states. Hence, in contradiction to proposals by other authors, no entanglement witness can be constructed solely by comparing the attainable uncertainties of entangled and separable states. However, our result gives rise to a huge simplification for computing global uncertainty bounds as they now can be deduced from local ones.
Furthermore, we provide the natural generalization of the Maassen and Uffink inequality for linear uncertainty relations with arbitrary positive coefficients.

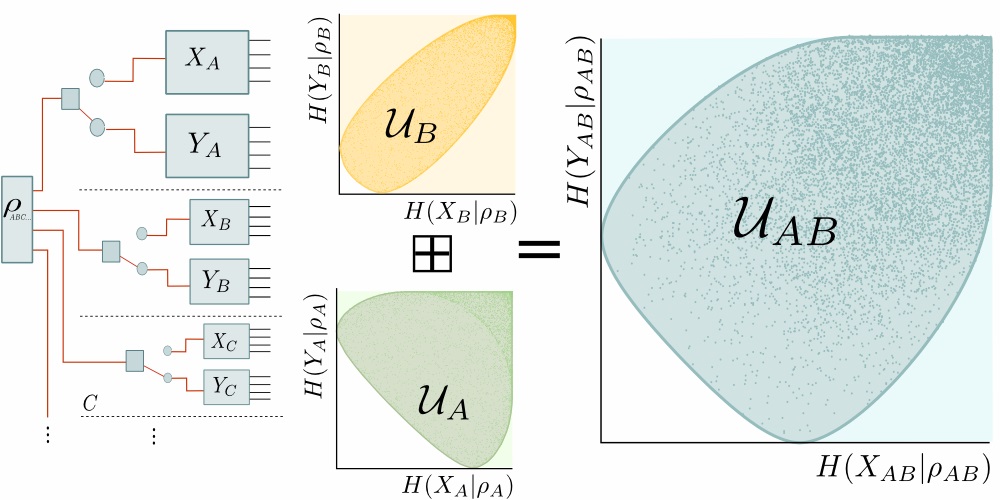
Popular summary

For pairs of local measurements performed on multipartite systems, it is shown that the optimal bound in any linear uncertainty relation, formulated in terms of Shannon entropies, is additive. This directly implies that minimal uncertainty can always be realized by fully separable states. Hence, we have an example for a task which is not improved by the use of entanglement. On the other hand, this gives rise to a huge simplification to the structure of global uncertainty bounds as they now can be deduced from local ones by a single letter formula. Furthermore, an extension of the Maassen and Uffink bound for arbitrary linear relations, with positive coefficients, is provided.

► BibTeX data

@article{Schwonnek2018additivityof,
  doi = {10.22331/q-2018-03-30-59},
  url = {https://doi.org/10.22331/q-2018-03-30-59},
  title = {Additivity of entropic uncertainty relations},
  author = {Schwonnek, Ren{\'{e}}},
  journal = {{Quantum}},
  issn = {2521-327X},
  publisher = {{Verein zur F{\"{o}}rderung des Open Access Publizierens in den Quantenwissenschaften}},
  volume = {2},
  pages = {59},
  month = mar,
  year = {2018}
}

► References

[1] J. Schneeloch, C. J. Broadbent, S. P. Walborn, E. G. Cavalcanti, and J. C. Howell. Einstein-Podolsky-Rosen steering inequalities from entropic uncertainty relations. Physical Review A, 87: 062103, 2013. 10.1103/PhysRevA.87.062103. arXiv:1303.7432.
https://doi.org/10.1103/PhysRevA.87.062103
arXiv:1303.7432

[2] A. C. Costa Sprotte, R. Uola, and O. Gühne. Steering criteria from general entropic uncertainty relations. 2017. arXiv:1710.04541.
arXiv:1710.04541

[3] A. Riccardi, C. Macchiavello, and L. Maccone. Multipartite steering inequalities based on entropic uncertainty relationss. 2017a. arXiv:1711.09707.
arXiv:1711.09707

[4] Z.-A. Jia, Y.-C. Wu, and G.-C. Guo. Characterizing nonlocal correlations via universal uncertainty relations. Phys. Rev. A, 96: 032122, 2017. 10.1103/PhysRevA.96.032122. arXiv:1705.08825.
https://doi.org/10.1103/PhysRevA.96.032122
arXiv:1705.08825

[5] M. Berta, M. Christandl, R. Colbeck, J. M. Renes, and R. Renner. The uncertainty principle in the presence of quantum memory. Nature Phys., 2010. 10.1038/nphys1734. arXiv:0909.0950.
https://doi.org/10.1038/nphys1734
arXiv:0909.0950

[6] F. Furrer, T. Franz, M. Berta, A. Leverrier, V. B Scholz, M. Tomamichel, and R. F Werner. Continuous variable quantum key distribution: Finite-key analysis of composable security against coherent attacks. Phys. Rev. Lett., 109: 100502, 2012. 10.1103/PhysRevLett.109.100502. arXiv:1112.2179.
https://doi.org/10.1103/PhysRevLett.109.100502
arXiv:1112.2179

[7] W. Heisenberg. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys., 43: 172–198, 1927. 10.1007/BF01397280.
https://doi.org/10.1007/BF01397280

[8] E. H. Kennard. Zur Quantenmechanik einfacher Bewegungstypen. Z. Phys., 44: 326–352, 1927. 10.1007/BF01391200.
https://doi.org/10.1007/BF01391200

[9] H. P. Robertson. The uncertainty principle. Phys. Rev., 34: 163–164, 1929. 10.1103/PhysRev.34.163.
https://doi.org/10.1103/PhysRev.34.163

[10] R. Schwonnek, L. Dammeier, and R.F. Werner. State-independent uncertainty relations and entanglement detection in noisy systems. Phys. Rev. Lett., 119: 170404, 2017. 10.1103/PhysRevLett.119.170404. arXiv:1705.10679.
https://doi.org/10.1103/PhysRevLett.119.170404
arXiv:1705.10679

[11] L. Dammeier, R. Schwonnek, and R.F. Werner. Uncertainty relations for angular momentum. New J. Phys., 9 (17): 093946, 2015. 10.1088/1367-2630/17/9/093046. arXiv:1505.00049.
https://doi.org/10.1088/1367-2630/17/9/093046
arXiv:1505.00049

[12] P. J. Coles and M. Piani. Improved entropic uncertainty relations and information exclusion relations. Phys. Rev. A, 89, 2014. 10.1103/PhysRevA.89.022112. arXiv:1307.4265.
https://doi.org/10.1103/PhysRevA.89.022112
arXiv:1307.4265

[13] S. Wehner and A. Winter. Entropic uncertainty relations – a survey. New J. Phys., 12: 025009, 2010. 10.1088/1367-2630/12/2/025009. arXiv:0907.3704.
https://doi.org/10.1088/1367-2630/12/2/025009
arXiv:0907.3704

[14] A. E. Rastegin. Rényi formulation of the entropic uncertainty principle for POVMs. J. Phys. A, 43: 155302, 2010. 10.1088/1751-8113/43/15/155302.
https://doi.org/10.1088/1751-8113/43/15/155302

[15] Y. Xiao, C Guo, F Meng, N. Jing, and M.-H. Yung. Incompatibility of observables as state-independent bound of uncertainty relations. 2017. arXiv:1706.05650.
arXiv:1706.05650

[16] P. Busch, P. Lahti, and R. F. Werner. Measurement uncertainty relations. J. Math. Phys., 55: 042111, 2014. 10.1063/1.4871444. arXiv:1312.4392.
https://doi.org/10.1063/1.4871444
arXiv:1312.4392

[17] R. Schwonnek, D. Reeb, and R. F. Werner. Measurement uncertainty for finite quantum observables. Mathematics, 4 (2): 38, 2016. 10.3390/math4020038. arXiv:1604.00382.
https://doi.org/10.3390/math4020038
arXiv:1604.00382

[18] J. M. Renes, V. B. Scholz, and S. Huber. Uncertainty relations: An operational approach to the error-disturbance tradeoff. Quantum, 1 (20), 2016. 10.22331/q-2017-07-25-20. arXiv:1612.02051.
https://doi.org/10.22331/q-2017-07-25-20
arXiv:1612.02051
https://arxiv.org/abs/1612.02051

[19] A. A. Abbott and C. Branciard. Noise and disturbance of qubit measurements: An information-theoretic characterization. Phys. Rev. A, 94: 062110, 2016. 10.1103/PhysRevA.94.062110. arXiv:1607.00261.
https://doi.org/10.1103/PhysRevA.94.062110
arXiv:1607.00261

[20] A. Barchielli, M. Gregoratti, and A. Toigo. Measurement uncertainty relations for discrete observables: Relative entropy formulation. Comm. Math. Phys., (357): 1253–1304, 2016. 10.1007/s00220-017-3075-7. arXiv:1608.01986.
https://doi.org/10.1007/s00220-017-3075-7
arXiv:1608.01986

[21] H. Maassen and J. B. M. Uffink. Generalized entropic uncertainty relations. Phys. Rev. Lett., 60: 1103–1106, 1988. 10.1103/PhysRevLett.60.1103.
https://doi.org/10.1103/PhysRevLett.60.1103

[22] R. F. Werner. Quantum states with einstein-podolsky-rosen correlations admitting a hidden-variable model. Phy. Rev. A, 40 (8): 4277, 1989. 10.1103/PhysRevA.40.4277.
https://doi.org/10.1103/PhysRevA.40.4277

[23] S. Friedland, V. Gheorghiu, and G. Gour. Universal uncertainty relations. Phys. Rev. Lett., 111 (23): 230401, 2013. 10.1103/PhysRevLett.111.230401. arXiv:1304.6351.
https://doi.org/10.1103/PhysRevLett.111.230401
arXiv:1304.6351

[24] A. Einstein, B. Podolsky, and N. Rosen. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev., 47 (10): 777, 1935. 10.1103/PhysRev.47.777.
https://doi.org/10.1103/PhysRev.47.777

[25] E. Schrödinger. Die gegenwärtige Situation in der Quantenmechanik. Naturwiss., 23 (48): 807–812, 1935. 10.1007/BF01491891.
https://doi.org/10.1007/BF01491891

[26] H.M. Wiseman, S. J. Jones, and A. C. Doherty. Steering, entanglement, nonlocality, and the Einstein-Podolsky-Rosen paradox. Phys. Rev. Lett., 98 (14): 140402, 2007. 10.1103/PhysRevLett.98.140402. arXiv:quant-ph/ 0612147.
https://doi.org/10.1103/PhysRevLett.98.140402

[27] G. Sharma, C. Mukhopadhyay, S. Sazim, and A.K. Pati. Quantum uncertainty relation based on the mean deviation. and arXiv:1801.00994.

[28] D. Deutsch. Uncertainty in quantum measurements. Phys. Rev. Lett., 50: 631–633, 1983. 10.1103/PhysRevLett.50.631.
https://doi.org/10.1103/PhysRevLett.50.631

[29] C. E. Shannon. A mathematical theory of communication. The Bell System Technical Journal, 27 (3): 379–423, 1948. 10.1002/j.1538-7305.1948.tb01338.x.
https://doi.org/10.1002/j.1538-7305.1948.tb01338.x

[30] A.N. Kolmogorov. On tables of random numbers. Theoretical Computer Science, 207 (2): 387–395, 1998. 10.1016/S0304-3975(98)00075-9.
https://doi.org/10.1016/S0304-3975(98)00075-9

[31] Hmolpedia. The Neumann-Shannon anecdote. http://www.eoht.info/page/Neumann-Shannon+anecdote.
http://www.eoht.info/page/Neumann-Shannon+anecdote

[32] M. Tribus and E. C. Mc Irvine. Energy and information. Sc. Am., 224: 178–184, 1971. 10.1038/scientificamerican0971-179.
https://doi.org/10.1038/scientificamerican0971-179

[33] F. Rozpędek, J. Kaniewski, P. J. Coles, and S. Wehner. Quantum preparation uncertainty and lack of information. New Journal of Physics, 19 (2): 023038, 2016. 10.1088/1367-2630/aa5d64. arXiv:1606.05565.
https://doi.org/10.1088/1367-2630/aa5d64
arXiv:1606.05565

[34] K. Abdelkhalek, R. Schwonnek, H. Maassen, F. Furrer, J. Duhme, P. Raynal, B.G. Englert, and R.F. Werner. Optimality of entropic uncertainty relations. Int. J. Quant. Inf., 13 (06): 1550045, 2015. 10.1142/S0219749915500458. arXiv:1509.00398.
https://doi.org/10.1142/S0219749915500458
arXiv:1509.00398

[35] H. Hadwiger. Minkowskische Addition und Subtraktion beliebiger Punktmengen und die Theoreme von Erhard Schmidt. 10.1007/BF01175656. Math.Z., 53 (3): 210–218, 1950.
https://doi.org/10.1007/BF01175656

[36] H. F. Hofmann and S. Takeuchi. Violation of local uncertainty relations as a signature of entanglement. Phys.Rev. A., 68: 032103, 2003. 10.1103/PhysRevA.68.032103. arXiv:quant-ph/0212090.
https://doi.org/10.1103/PhysRevA.68.032103
arXiv:quant-ph/0212090

[37] O. Gühne. Detecting quantum entanglement: entanglement witnesses and uncertainty relations. PhD thesis, Universität Hannover, 2004. URL http://d-nb.info/972550216.
http://d-nb.info/972550216

[38] B. Lücke, J. Peise, G. Vitagliano, J. Arlt, L. Santos, G. Tóth, and C. Klempt. Detecting multiparticle entanglement of Dicke states. Phys. Rev. Lett., 112: 155304, 2014. 10.1103/PhysRevLett.112.155304. arXiv:1403.4542.
https://doi.org/10.1103/PhysRevLett.112.155304
arXiv:1403.4542

[39] O. Gühne and G. Tóth. Entanglement detection. Phys. Rep., 474 (1–6): 1 – 75, 2009. ISSN 0370-1573. 10.1016/j.physrep.2009.02.004.
https://doi.org/10.1016/j.physrep.2009.02.004

[40] O. Gühne and A. Costa. Private communication, 2017.

[41] I. Białynicki-Birula and J. Mycielski. Uncertainty relations for information entropy in wave mechanics. Communications in Mathematical Physics, 44 (2): 129–132, 1975. 10.1007/BF01608825.
https://doi.org/10.1007/BF01608825

[42] W. Beckner. Inequalities in Fourier analysis. Annals of Mathematics, pages 159–182, 1975. 10.2307/1970980.
https://doi.org/10.2307/1970980

[43] I. I. Hirschman. A note on entropy. American Journal of Mathematics, 79 (1): 152–156, 1957. 10.2307/1970980.
https://doi.org/10.2307/1970980

[44] H. Maassen. The discrete entropic uncertainty relation. Talk given in Leyden University. Slides of a later version available from the author's website, 2007.

[45] H. Maassen. Discrete entropic uncertainty relation. Springer, 1990. 10.1007/BFb0085519. `Quantum Probability and Applications V' (Proceedings Heidelberg 1988),Lecture Notes in Mathematics 1442.
https://doi.org/10.1007/BFb0085519

[46] A. Rényi. On measures of entropy and information. Fourth Berkeley Symposium on Mathematical Statistics and Probability, pages 547–561, 1961.

[47] J. Hendrickx and A. Olshevsky. Matrix p-norms are NP-hard to approximate if $p\neq 1, 2, \infty$. SIAM J. M. A. A., 31: 2802–2812, 01 2010. 10.1137/09076773X. arXiv:0908.1397.
https://doi.org/10.1137/09076773X
arXiv:0908.1397

[48] J. Rohn. Computing the norm $\Vert A \Vert_{\infty,1}$, is NP-hard. Lin. and Multilin. Alg., 47 (3): 195–204, 2000. 10.1080/03081080008818644.
https://doi.org/10.1080/03081080008818644

[49] K. Drakakis and B. A. Pearlmutter. On the calculation of the $l_2 \rightarrow l_1$ induced matrix norm. Int. J. Alg., 3 (5): 231–240, 2009.

[50] M. Riesz. Sur les maxima des formes bilinéaires et sur les fonctionnelles linéaires. Acta Mathematica, 49 (3-4): 465–497, 1926. 10.1007/BF02564121.
https://doi.org/10.1007/BF02564121

[51] O. G. Thorin. Convexity theorems generalizing those of M. Riesz and Hadamard with some applications. 1948.

[52] S. Golden. Lower bounds for the Helmholz function. Phys. Rev, 137: B1127–B1128, 1965. 10.1103/PhysRev.137.B1127.
https://doi.org/10.1103/PhysRev.137.B1127

[53] C. J. Thompson. inequality with applications in statistical mechanics. J. Math. Phys., 6 (11): 1812–1813, 1965. 10.1063/1.1704727.
https://doi.org/10.1063/1.1704727

[54] P. J. Forrester and C. J. Thompson. The Golden-Thompson inequality: Historical aspects and random matrix applications. J. Math. Phys., 55 (2): 023503, 2014. 10.1063/1.4863477. arXiv:1408.2008.
https://doi.org/10.1063/1.4863477
arXiv:1408.2008

[55] T. Tao. The Golden-Thompson inequality| What's new?, 2010. https://terrytao.wordpress.com/2010/07/15/the-golden-thompson-inequality/.
https://terrytao.wordpress.com/2010/07/15/the-golden-thompson-inequality/

[56] R. Frank and E. Lieb. Entropy and the Uncertainty Principle. Ann. l'Ins. Henri Poincare, 13 (8): 1711–1717, 2012. 10.1007/s00023-012-0175-y. arXiv:1109.1209.
https://doi.org/10.1007/s00023-012-0175-y
arXiv:1109.1209

[57] E.H. Lieb. Convex trace functions and the Wigner-Yanase-Dyson conjecture. Adv. in Math., 11: 267–288, 1973. 10.1016/0001-8708(73)90011-X.
https://doi.org/10.1016/0001-8708(73)90011-X

[58] M. Berta D. Sutter and M. Tomamichel. Multivariate trace inequalitie. Com. Mat. Phys., 352 (1): 37–58, 2016. 10.1007/s00220-016-2778-5. arXiv:1604.03023.
https://doi.org/10.1007/s00220-016-2778-5
arXiv:1604.03023

[59] M. Lemm. On multivariate trace inequalities of Sutter, Berta and Tomamichel. J. Mat. Phys., 59: 012204, 2018. 10.1063/1.5001009. arXiv:1708.04836.
https://doi.org/10.1063/1.5001009
arXiv:1708.04836

[60] F. Hansen. Multivariate extensions of the Golden- Thompson inequality. An. Func. An., 6(4): 301–310, 2015. 10.15352/afa/06-4-301. arXiv:1406.5686.
https://doi.org/10.15352/afa/06-4-301
arXiv:1406.5686

[61] W. Grey and G. Sinnamon. Product operators on mixed norm spaces. Lin. and Non. Lin. A., 2 (2): 189–197, 2016. 10.1016/j.jmva.2017.09.008. arXiv:1602.0879.
https://doi.org/10.1016/j.jmva.2017.09.008
arXiv:1602.0879

[62] G. H. Hardy, J.E. Littlewood, and G. Polya. Inequalities. Cambridge University Press, 1934.

[63] M. A. Ballester and S. Wehner. Entropic uncertainty relations and locking: tight bounds for mutually unbiased bases. Phys. Rev. A, 75, 2007. 10.1103/PhysRevA.75.022319. arXiv:quant-ph/0606244.
https://doi.org/10.1103/PhysRevA.75.022319
arXiv:quant-ph/0606244

[64] A. Winter. Weak locking capacity of quantum channels can be much larger than private capacity. Journal of Cryptology, 30 (1): 1–21, 2017. 10.1007/s00145-015-9215-3. arXiv:1403.6361.
https://doi.org/10.1007/s00145-015-9215-3
arXiv:1403.6361

[65] J. Sánches-Ruiz. Optimal entropic uncertainty relation in two-dimensional Hilbert space. Phys. Lett. A, 244: 189–195, 1998. 10.1016/S0375-9601(98)00292-8.
https://doi.org/10.1016/S0375-9601(98)00292-8

[66] J. Sánchez-Ruiz. Improved bounds in the entropic uncertainty and certainty relations for complementary observables. Phys. Lett. A, 201: 125–131, 1995. 10.1016/0375-9601(95)00219-S.
https://doi.org/10.1016/0375-9601(95)00219-S

[67] A. Riccardi, C. Macchiavello, and L. Maccone. Tight entropic uncertainty relations for systems with dimension three to five. Phys. Rev. A, 95: 032109, 2017b. 10.1103/PhysRevA.95.032109. arXiv:1701.04304.
https://doi.org/10.1103/PhysRevA.95.032109
arXiv:1701.04304

[68] T. Simnacher and N. Wyderka. Private communication, 2017.

Cited by

[1] Tamás Kriváchy, Florian Fröwis, and Nicolas Brunner, "Tight steering inequalities from generalized entropic uncertainty relations", Physical Review A 98 6, 062111 (2018).

[2] Qing-Hua Zhang and Shao-Ming Fei, "Entropic uncertainty relations with quantum memory in a multipartite scenario", Physical Review A 108 1, 012211 (2023).

[3] Bo-Fu Xie, Fei Ming, Dong Wang, Liu Ye, and Jing-Ling Chen, "Optimized entropic uncertainty relations for multiple measurements", Physical Review A 104 6, 062204 (2021).

[4] Antonio F. Rotundo and René Schwonnek, "Entropic uncertainty principle for mixed states", Physical Review Research 6 3, 033043 (2024).

[5] Yuan-Yuan Zhao, Guo-Yong Xiang, Xiao-Min Hu, Bi-Heng Liu, Chuan-Feng Li, Guang-Can Guo, René Schwonnek, and Ramona Wolf, "Entanglement Detection by Violations of Noisy Uncertainty Relations: A Proof of Principle", Physical Review Letters 122 22, 220401 (2019).

[6] René Schwonnek, Koon Tong Goh, Ignatius W. Primaatmaja, Ernest Y.-Z. Tan, Ramona Wolf, Valerio Scarani, and Charles C.-W. Lim, "Device-independent quantum key distribution with random key basis", Nature Communications 12 1, 2880 (2021).

[7] Alberto Riccardi, Giovanni Chesi, Chiara Macchiavello, and Lorenzo Maccone, "Tight Bounds from Multiple‐Observable Entropic Uncertainty Relations", Annalen der Physik 536 8, 2400020 (2024).

[8] Ana Costa, Roope Uola, and Otfried Gühne, "Entropic Steering Criteria: Applications to Bipartite and Tripartite Systems", Entropy 20 10, 763 (2018).

[9] Timo Simnacher, Nikolai Wyderka, René Schwonnek, and Otfried Gühne, "Entanglement detection with scrambled data", Physical Review A 99 6, 062339 (2019).

[10] Mario Berta, David Sutter, and Michael Walter, "Quantum Brascamp–Lieb Dualities", Communications in Mathematical Physics 401 2, 1807 (2023).

The above citations are from Crossref's cited-by service (last updated successfully 2024-09-01 02:12:09) and SAO/NASA ADS (last updated successfully 2024-09-01 02:12:10). The list may be incomplete as not all publishers provide suitable and complete citation data.

This Paper is published in Quantum under the Creative Commons Attribution 4.0 International (CC BY 4.0) license. Copyright remains with the original copyright holders such as the authors or their institutions.